gasilbalance.blogg.se

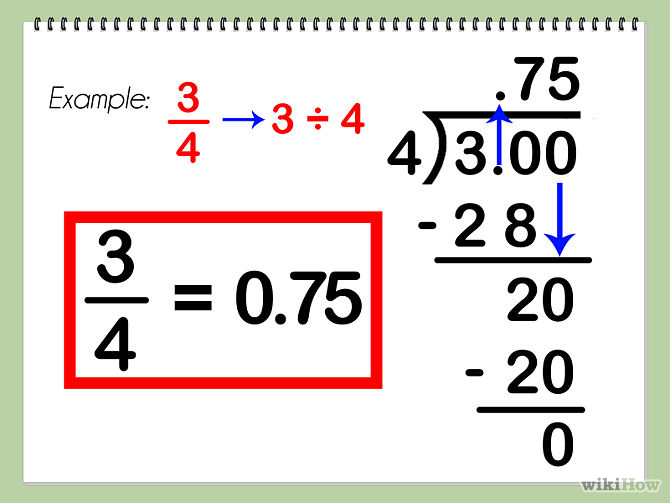
We're moving the decimal two to the right, one,Īnd on and on and on. Them are repeating, we only want to multiplyīy 10 to the second power. Situation, even though we have three numbersīehind the decimal point, because only two of So that the repeating pattern can be right under itself Multiply this by 1,000x to get the decimal all theĭon't want to do that. Let's say we have the numberĠ.714, and the 14 is repeating. So 0.363636 forever andįorever repeating is 4/11. And both the numerator and theĭenominator is divisible by 9, so we can reduce this. Subtract x from 100x, the repeating parts And notice when we multiplied byġ00x, the 3's and the 6's still line up with each other Going to be 36.363636 on and on and on forever. To- the decimal is going to be there now, so it's So 100x is going toīe equal to what? We're shifting this two Two to the right, we have to have multiplied byġ00 or 10 to the second power. So that when we line them up, the decimal parts will still It one over- we want to shift it over enough Multiplying it by 10- 10 would only shift In the last video, we set this as equal to x. Since the bar's over the 3 and the 6, both of So let's say I hadĠ.36 repeating, which is the same thing as 0 point. Multiple digits repeating on and on forever. Something a little bit more interesting, which is

Repeating on and on forever, and we were able to convert We did some examples where we had one digit Two digits (67) repeat, and three digits after the decimal point (413) are not part of the repeating group. To create the numerator, we subtract the number formed by decimal digits not part of the repeating group (if any), from the number formed by the decimal digits up to and including the last digit of the first occurrence of the repeating group.Įxample: convert 0.4136767. To create the denominator, we use a digit 9 for every digit in the repeating group, then we add a digit 0 to the right for every digit after the decimal point not part of the repeating group (if any). For this answer, we will consider just repeating decimals between 0 and 1 (if the repeating decimal is greater than 1 or negative, we can convert the part after the decimal point to a fraction and so make a mixed number, negative fraction, or negative mixed number.) Good question! Yes, there’s an alternative method.